Verification of Numerical Models
Dr. Robin Patrick Mooney
Email: [javascript protected email address]
Tel: +353 1 896 2396
Dr. Robin Patrick Mooney
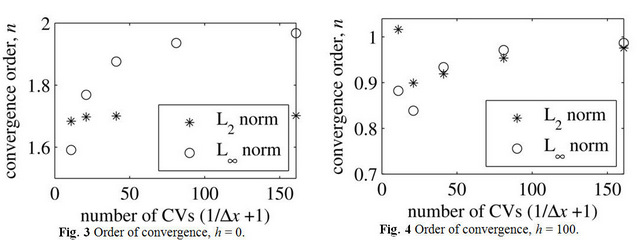
DescriptionVerification in numerical modelling is too often overlooked. As models become increasingly complicated, modellers avoid the verification step of model development because of its perceived difficulty (citing a lack of suitable analytical models) in favour of model validation with experimental results. However, there is a consensus in the literature, over a diverse range of Science and Engineering topics, that model verification is an important and worthwhile prerequisite to model validation; for example in; Computational Fluid Dynamics (CFD) [1], Solidification modelling [2], Biomechanics modelling [3], Civil Engineering modelling [4], and in Multiscale modelling [5]. Verification of a numerical model is complete when it can be demonstrated that the governing partial differential equation (PDE) being modelled is solved correctly. More specifically, this is when the error observed between the exact PDE solution and the numerical results is due to truncation error arising by discretisation only, and when the order of this error matches the theoretical order accuracy of the discretisation method. Validation is distinguished from verification as the process by which a model is demonstrated to fit the physical phenomena being modelled through comparison of numerical results with experimental data. In short, according to Boehm [6] and Blottner [7], verification is “solving the equations right” and validation is “solving the right equations”. A convergence study can be used to verify calculations in a numerical model where the order of error, p (known as the observed order of accuracy), is calculated using simulation results at two or more different grid resolutions. If a model is claimed to have nth order accuracy (i.e., it uses nth order discretisation); one would expect the observed error in subsequent simulations of a grid convergence study to be proportional to n. When it can be shown that p nearly equal to n, the model is then verified [8]. Pelletier and Roache [9] subdivide Verification into two subjects: Verification of Code and Verification of Calculations. The former involves error evaluation by comparing numerical results with an exact solution at two different grid resolutions to calculate p; the latter involves error estimation using grid convergence studies, in this case, p can be calculated using numerical results at three grid resolutions. The ‘Verification of Calculations’ method (originally proposed by DeVahl Davis [10]) for extracting the observed order of accuracy from a numerical scheme is utilised. The main advantage of this method is that it does not require an exact, analytical solution of the governing PDE.
References[1] D. Pelletier, -Ccedil, . Turgeon, D. Lacasse, and J. Borggaard, “Adaptivity, Sensitivity, and Uncertainty: Toward Standards of Good Practice in Computational Fluid Dynamics,” AIAA J., May 2012.
[2] M. Založnik, S. Xin, and B. Šarler, “Verification of a numerical model of macrosegregation in direct chill casting,” Int. J. Numer. Methods Heat Fluid Flow, vol. 18, no. 3/4, pp. 308–324, May 2008.
[3] H. B. Henninger, S. P. Reese, A. E. Anderson, and J. A. Weiss, “Validation of computational models in biomechanics,” Proc. Inst. Mech. Eng. Part H J. Eng. Med., vol. 224, no. 7, pp. 801–812, Jul. 2010.
[4] J. Carpenter, J. Wicks, B. Clarke, A. Borthwick, and R. Falconer, “The importance of understanding computer analyses in civil engineering,” Proc. ICE - Civ. Eng., vol. 166, no. 3, pp. 137–143, Aug. 2013.
[5] A. Hoekstra, B. Chopard, and P. Coveney, “Multiscale modelling and simulation: a position paper.,” Philos. Trans. A. Math. Phys. Eng. Sci., vol. 372, no. 2021, p. 20130377–, Aug. 2014.
[6] B. W. Boehm, Software Engineering Economics. New Jersey: Prentice-Hall, 1981.
[7] F. G. Blottner, “Accurate Navier-Stokes results for the hypersonic flow over a spherical nosetip,” J. Spacecr. Rockets, vol. 27, no. 2, pp. 113–122, Mar. 1990.
[8] P. J. Roache, “Verification of Codes and Calculations,” AIAA J., vol. 36, no. 5, pp. 696–702, May 1998.
[9] D. Pelletier and P. J. Roache, “Verification and Validation of Computational Heat Transfer,” in Handbook of Numerical Heat Transfer, 2nd ed., W. J. Minkowycz, E. M. Sparrow, and J. Y. Murty, Eds. John Wiley & Sons, Inc., 2000, pp. 417–442.
[10] G. De Vahl Davis, “Natural convection of air in a square cavity: A bench mark numerical solution,” Int. J. Numer. Methods Fluids, vol. 3, no. 3, pp. 249–264, May 1983.

